The Mass of the Local Supercluster

As we've discussed in class, there are deviations from the Hubble flow of galaxies known as peculiar velocities. At late times in the Universe's history these peculiar motions are due purely to gravitaional accelerations from asymmetric mass distributions on relatively small scales. The Local Group of galaxies sits at the edge of what is known as the Local Supercluster (see Figure 3) which is dominated by the Virgo cluster. The Local Group is being gravitationally pulled toward the Virgo cluster, this is called the Virgocentric infall.

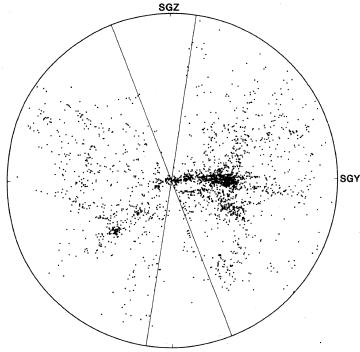
Figure 3: The distribution of 2175 bright galaxies, out to roughly 50 Mpc. The Local Supercluster extends to the right, with the Milky Way (at center) located near the edge of the supercluster. The plane of the Milky Way bisects the ``empty'' slices; galaxies within the slices are hidden from view by Galactic dust and gas (the zone of avoidance). (Figure from Tully, Ap. J., 257, 389, 1982.)

1. The giant elliptical galaxy M87 sits at the center of the Virgo cluster and is a good galaxy to use to estimate the value of the Virgocentric infall velocity (it should have no line of site peculiar velocity due to virial motions within the cluster). Its redshift is measured to be z = 0.00314 in the frame of the Local Group. Calculate its peculiar velocity, 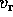, assuming that the distance to the Virgo cluster is 16 Mpc, and .
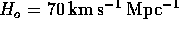2. Now, we can write the infall velocity in terms of the gravitational acceleration

   

   What time would we want to use? Write 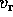in terms of G, the total mass of the Local Supercluster, M, the distance to the cluster, d, and your expression for time.

3. Doing this analysis in all its hairy-backed-Kansas-mountain-goat-ugliness would yield the expression

   

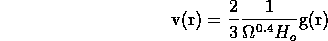
   where 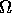is the cosmological density parameter. After completing Problem #3 of this assignment come back to this part and tell me why this expression kinda makes sense. Use 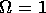and the previous values for 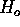, and distance, and also include your previous expression for g. Calculate the mass of the Local Supercluster (in solar masses) assuming that our motion relative to the cluster is not strongly disturbed by the tidal fields of more distant mass concentrations.

4. The total luminosity of the Local Supercluster is 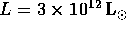. Compute the mass-to-light ratio (in units of M 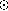/L 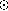) and explain the result.