


Next: Synodic versus Sidereal Periods
Up: No Title
Previous: Orbits of a Simple
The planet Mars is observed to move slowly eastward relative
to the fixed stars from night to night. Occasionally, however,
it appears to double back on its path, moving westward for
a few months. This is called a retrograde loop.
Consider the heliocentric model of Copernicus.
Assume, by way of approximation, that Mars and Earth describe
circular orbits, that these orbits are coplanar, and that
Mars is in opposition at time t=0, i.e. the Sun, Earth
and Mars lie along a line, in that order, at t=0.
Take 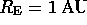 ,
,
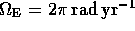 ,
and
,
and 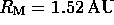 .
.
-
What is
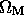 ?
?
-
Carefully (and roughly to scale, if possible) draw a diagram
of Copernicus's heliocentric system. By marking on it several
sequential positions of Mars and Earth, show that Mars,
as viewed from Earth, executes a retrograde loop against
the background of the fixed stars in a time interval about t=0.
-
Estimate approximately how long the retrograde loop lasts,
as viewed from Earth.
-
Calculate the time that elapses between successive retrograde
loops.
-
Calculate the angular separation between the locations of
successive retrograde loops, as viewed from Earth.
What would be the angular separation if Mars described a
different orbit with
 ? Comment.
? Comment.
Ptolemy devised a geocentric explanation of retrograde
loops involving epicycles.
-
Carefully (and roughly to scale, if possible) draw a diagram
of Ptolemy's geocentric system. By marking on it several
sequential positions of Mars and Earth, show how the
geocentric system leads to retrograde loops.
-
What features of real planetary motion (as opposed to the
idealised motion considered here) did Ptolemy's model
fail to explain economically?
In reality, the orbit of Mars is inclined with respect to
the plane of the Earth's orbit (the ecliptic) by an angle
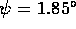 . This is shown in Fig. 1.
. This is shown in Fig. 1.
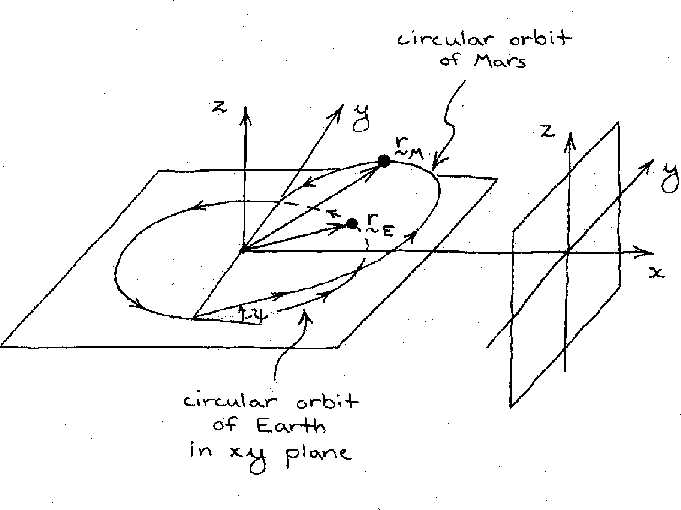
Figure 1:
-
Write down
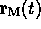 and
and 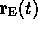 .
.
-
What is the relative position of Mars as seen from Earth?
-
Project this relative position onto the y-z plane of
the sky for
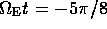 ,
, 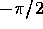 ,
, 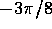 ,
, 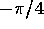 ,
, 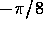 ,
0,
,
0, 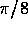 ,
, 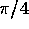 ,
, 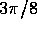 ,
, 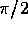 ,
, 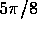 .
Graph the result neatly on ruled axes, appropriately scaled.
You should get a retrograde loop.
.
Graph the result neatly on ruled axes, appropriately scaled.
You should get a retrograde loop.



Next: Synodic versus Sidereal Periods
Up: No Title
Previous: Orbits of a Simple
Bryan J. Mendez
Fri Aug 27 17:24:54 PDT 1999
![]() ,
,
![]() ,
and
,
and ![]() .
.