Astronomy 10: Lecture 6

Lecture 6: Basic Physics

Reading Assignment: Arny pp. 69-73, Chpt. 2

Newton's Laws of Motion

In 1687 Netwon finally published his Principia were he laid out his laws fundamental laws of motion.
  1. The Law of Inertia: If the sum of the forces on an object add to zero then the object's velocity (speed + direction) will remain constant. This is sometimes stated as, "An object in motion tends to stay in motion, and an object rest tends to stay at rest." The idea here is simple, do nothing to an object and it will keep doing what it has been doing all along. The idea of inertia is that an object will resist a change in its motion (either making it move or trying to stop it from moving). Note that since neither speed nor direction of motion changes that we always have motion in a straight line when there are no forces.

  2. F = ma : This law states that the acceleration (change in velocity, which can be a change in speed, direction, or both) experienced by an object is directly proportional to the amount of force exerted on it. The constant of proportionality is the object's mass. This is how mass is essentially defined. Sometimes it's refered to as inertial mass.

  3. Action and Reaction: This law states that when two bodies interact, they create equal and opposite forces on each other. (Examples: Two gravitating bodies exerting the same gravitational force on each other, two skateboarders pushing on one another, a ball bouncing off the wall, astronaut throwing a hammer, etc...)

Circular Motion

The law of inertia tells us that if there are no forces acting on an object then its motion is in a straight line. So how do we produce circular motion, like that of the planets in their orbits? We have to apply a force. The force that we need to apply must constantly change the direction of of the object's motion without altering the tangential speed of the object. We can show this with an object tied to the end of a string. If we swing the object around in a circle above our heads the force that is causing the object to go around is the tension in the string. You can feel the tension as you swing the the object. If we were to suddenly cut the string the object would continue off on a tangential line from the spot it was released. It will not continue on in a curved path.

(DEMO)

The force that keeps planets, moons, and whatnot in orbits is gravity, the natural attraction of all matter to other matter. We can visualize how to put something in orbit by imagining firing a canonball from a very high mountain. The greater the initial velocity we give the ball the farther down range it will go before falling to the Earth. If we get it at just the right speed it will continue to fall toward the Earth, but the Earth's curvature will continue to curve away from it and it will make a full circle and eventually hit the back end of the canon.

So an object in orbit is indeed experiencing gravity. For example the Space Shuttle when it is in orbit around the Earth is always falling toward the Earth, but it is also heading tangentially at just the right speed to stay in a circle (or ellipse). So it is always under the influence of gravity. The astronauts appear weightless because they and the Shuttle are all falling together at the same rate.

The Universal Law of Gravity

Newton made the connection between the "magnetic" force that Kepler had supposed caused his 2nd law implied and the everyday force of gravity. The old story goes that one day Newton was out sitting under an apple tree looking at the Moon. The story goes that when he saw an apple fall he wondered if the force that was keeping the Moon in orbit around the Earth was the same force that made the apple fall to the ground. That would mean that the Moon is always falling toward Earth in the way we described above. He also then reasoned that it must be gravity that holds the Earth and planets in orbit about the Sun. So we are always falling toward the Sun. He studied Kepler's 3rd law of planetary motion and his own laws of motion to come up with a Universal Law of Gravitation. Given two masses, m1 and m2 the force between them is given by
F = - Gm1m2/r2
Where r is the distance between the two objects and G is a constant found empirically, G = 6.67 x 10-11 m3*g-1*s-2. By Netwon's 3rd law this is the force felt by both objects. The negative sign indicates that the force is attractive it draws the two bodies together. For example the force of attracttion that your body feels toward the Earth is the same force of attraction that the Earth feels toward you. But since Earth is way more massive than you its acceleration is much, much, much less than yours. You can see this by relating the law of gravitation to Newton's second law.
F = - GMmyou/r2 = - myouayou = -Ma
So your acceleration toward Earth is
ayou = GM/r2
And the Earth's acceleration toward you is
a = Gmyou/r2
And so the ratio of Earth's acceleration to yours is
a/ayou = myou/M
Which is a very small number. And so Earth doesn't react much to your presence, but you react alot to Earth's.

Kepler's 3rd Law

Netwon also found that he could derive Kepler's 3rd law using his new fangled-dangled invention: calculus. In doing so, he realized that he could figure out what that constant of proportionality was in Kepler's 3rd Law. It turns out that it depends on the masses of the two objects in question. He found the general form to be
P2 = 42/G(m1 + m2) * a3
So the constant k = 42/G(m1 + m2).

IMPORTANT: If m2 << m1 then we may ignore m2 and then the relation is just

P2 = 42/Gm1 * a3
This is the case for all the planets in the solar system compared to the mass of the Sun and likewise for most of the moons in the solar system compared to their mother planets.

This fact can be used to measure the mass of the Sun. For all planets in the Solar System we may write

P2 = 42/GM * a3
Let's rearrange the equation in the usual way to isolate the quantity that we want to know.
M = 42/G * a3/P2
We know all these values for Earth and if we plug them in we find that
M = 2 x 1030 kg

Energy

Now we come to a concept known as energy. Energy is defined as a quantity that measures the ability of a system to do work or cause motion. There are two kinds of mechanical energy
  1. Kinetic Energy: the energy of motion
    for any object with mass, m, and velocity, v, its kinetic energy, KE, is given by
    KE = 1/2 mv2

  2. Potential Energy: the amount of work that can potentially be done
    This has no simple definition, it is defined in a mathematical way. For an object, with mass, m, under the gravitational attraction of another with mass, M, and a distance, r, away the potential energy, PE, is
    PE = - GMm/r
The total mechanical energy, E, of a system is given by the sum of these two energies.
E = KE + PE

E is a constant and so KE and PE can only change in such a way that as one becomes smaller the other becomes larger by the same amount. In other words we can convert potential energy into kinetic energy and vice versa.

When you throw a ball up into the air, it initially has no Potential Energy, but has all Kinetic Energy. As the ball ascends it slows down, losing Kinetic Energy but gaining Potential Energy (the distance between the ball and Earth is increasing). When it reaches the top of its trajectory it has no velocity and therefore no Kinetic Energy, therefore it now has only Potential Energy. As the ball falls back to Earth, the situation reverses; Potential Energy converting to Kinetic Energy. All the while the value of E stays fixed.

Surface Gravity

Newton spent many extra years thinking about a certain problem involving the gravity of a spherically symmetric mass. He believed that the body would behave as though all of its mass was concentrated at the center. But in order to prove this he would need to invent integral calculus. Indeed his intuition was correct.

That means if you have mass, m, and are standing on the surface of a planet with Mass, M, and Radius, R, then the force of gravity acting on you is

F = -GMm/R2
.

Now notice also that it is true by Newton's 2nd Law that

F = -GMm/R2 = ma
Simplifying the expression...
a = -GM/R2
This says that the acceleration you feel due to the planet is independent of your mass. That's just what Galileo showed in his famous experiment of dropping canon balls from the leaning tower of Pisa.

(NOTE: the force you feel due to gravity is your weight)

Escape Velocity

If you wish to escape from the surface of a gravitating object there is a special velocity you must have in order to accomplish this. It's called the escape velocity. The best way to understand this is to think in terms of energy. In order to be able to escape from a planet you want to have an initial velocity that will make your total mechanical energy be unbound. Recall that the total mechanical energy is written as

E = KE + PE
In our initial system we have KE = 1/2 mvesc2, and PE = -GMm/R. We want E to at least be equal to zero to be unbound. So we may then write
E = 1/2 mvesc2 - GMm/R = 0
Let's now solve this for the escape velocity.
1/2 mvesc2 = GMm/R
So, again we see that the mass, m, of the object is unimportant to the calculation.
vesc = SQRT[2GM/R]

An interesting idea that was raised not long after Newton's time was that if an object were to have just enough mass enclosed in a small enough radius the escape velocity would be greater than the speed of light, and then not even light could escape the surface of this object. It was then known as a "Black Star". Today this is rather similar to the idea of a Black Hole.

Planetary Orbits

Planetary orbits were found by Kepler to be ellipses. But Newton found that they are even more general than ellipses, they are conic sections.
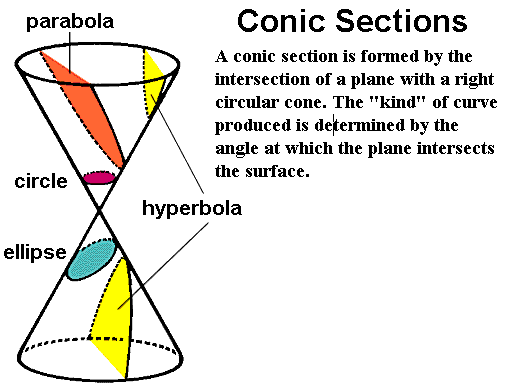Another way that these curves are thought of in relation to one another is in terms of eccentricity; it's the measure of the amount of squashiness a conic section has. A conic section with an eccentricity, e = 0, is a circle, 0 < e < 1 is an ellipse, e = 1 is a parabola, and e > 1 is a hyperbola.

The conic section that an orbit will exhibit depends on its total energy. If the total energy of the system is negative then the orbit is bound and is an ellipse. If the energy is exactly equal to zero then it is unbound and follows a parabolic shape. If the energy is positive, then the orbit is unbound and follows a hyperbola. This is summed up in the following table...

Energy Bound/Unbound Shape Eccentricity
E < 0, minimum Bound Circle e = 0
E < 0 Bound Ellipse 0 < e < 1
E = 0 Unbound Parabola e = 1
E > 0 Unbound Hyperbola e > 1

Return to Class Notes Page